Graph A Cube Root Function

Cube Root Function

The cube root function involves the cube root symbol ∛ (which stands for cube root) and hence let usa call up a few things nearly it. The cube root of a number 'a' is a number 'b' such that bⁱⁱⁱ = a. i.e., if b³ = a ⇒ b = ∛a. i.e., if 'b' is the cube of 'a' and so 'a' is the cube root of 'b'. Some examples are:

2ⁱⁱⁱ = viii ⇒ ∛8 = 2
(-2)³ = -8 ⇒ ∛(-eight) = -2
0³ = 0 ⇒ ∛0 = 0

Thus, the cube root of a positive number is positive and that of a negative number is negative. Let us use all these facts to understand the cube root function.

1.	What is Cube Root Function?
2.	Domain and Range of Cube Root Function
3.	Asymptotes of Cube Root Function
four.	Graphing Parent Cube Root Function
5.	Graphing Cube Root Functions
6.	Backdrop of Cube Root Function
vii.	FAQs on Cube Root Part

What is Cube Root Office?

The cube root function is the changed of the cubic function. We know that the parent cubic function is of the class f(x) = x³and this role is increasing, one-ane, and onto. Hence, it is a bijection. Thus, its inverse function, which is cube root function, is of the class f(10) = ∛x is as well a bijection. We know that a function and its changed function are symmetric with respect to the line y = 10 so the graphs of the parent cubic function and parent cube root functions look like this.

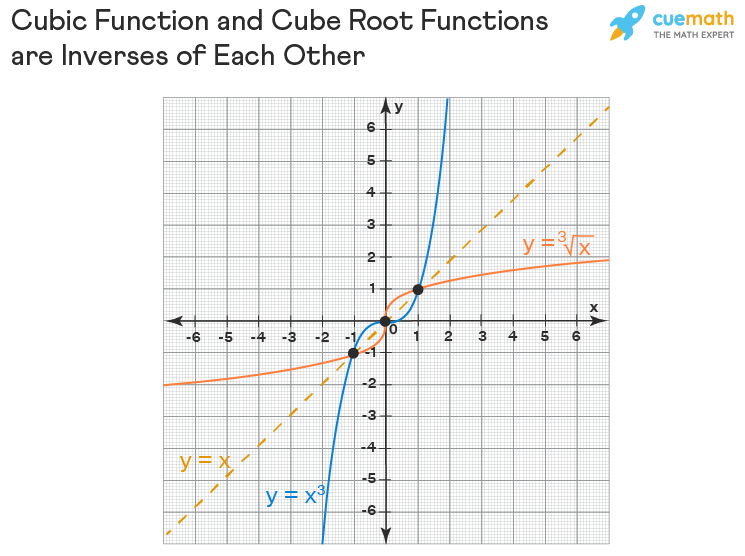
cube root function

f(x) = ∛x is the basic/parent cube root function. Just transformations may be applied on this function. Thus, the general form of cube root function is:

f(x) = a ∛(bx - h) + k

Here, a, b, h, and k are real numbers where 'h' represents the horizontal translation, 'k' represents the vertical translation, and 'a' and 'b' represent the dilations.

Domain and Range of Cube Root Function

We take already seen in the introduction that the cube root is defined for all numbers (positive, existent, and 0). Thus, for whatever cube root office f(10), there is no ten where f(x) is non defined. Thus, its domain is the set of all real numbers (R). In the same way, a cube root office results in all numbers (positive, existent, and 0), and hence its range is also the gear up of all real numbers. Thus, a cube root function is f(x): R → R. i.e.,

The domain of a cube root role is R.
The range of a cube root function is R.

Asymptotes of Cube Root Role

The asymptotes of a function are lines where a part of the graph is very close to those lines but information technology actually doesn't touch the lines. Allow us accept the parent cube root function f(x) = ∛x. Then

As 10 → ±∞, f(x) → ±∞ and hence information technology doesn't have horizontal asymptotes.
At that place is no 'ten' where f(x) is not defined and hence it doesn't have vertical asymptotes.

Thus, a cube root function doesn't have any asymptotes.

Graphing Parent Cube Root Office

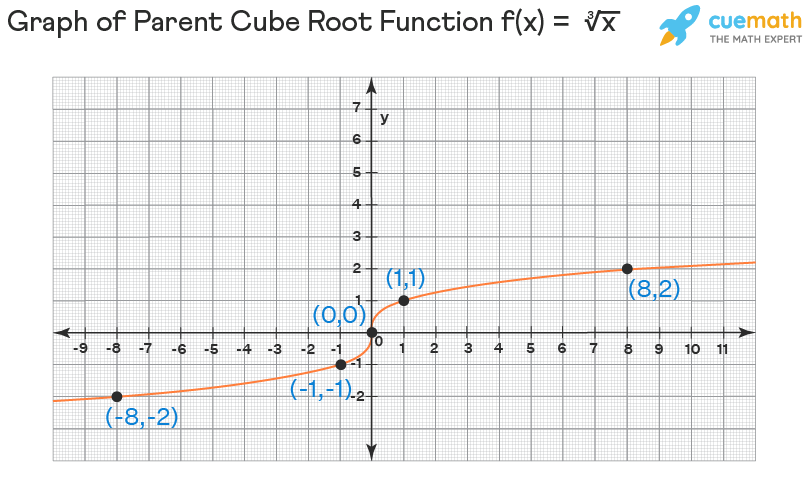
We have already seen that the domain and range of a cube root function is the set of all real numbers and it has no asymptotes. Allow us see how to graph the parent cubic root part f(10) = ∛x first and later on we will extend this process to graph any general cube root role. Let us construct a table of values with some random real numbers (as the domain is the set of all real numbers) for 10 (with some to exist positive, some to be negative, and besides a 0). Let us substitute each value in the part f(x) = ∛x to observe the corresponding y-value.

ten	y
-8	∛(-8) = -ii
-one	∛(-1) = -1
0	∛0 = 0
1	∛1 = 1
8	∛8 = 2

Here, we have chosen -8, -1, 0, 1, and 8 to be the 10 values as they are perfect cubes and they will assistance us to calculate y-values easily. Now, the points from the table are (-eight, -ii), (-1, -1), (0, 0), (1, 1), and (8, ii). Allow us plot all these points on the graph sheet and join them by a curve which gives the graph of the cube root office f(x) = ∛x. Also, do not forget to extend the graph on both sides.

graphing cube root function

Graphing Cube Root Functions

To graph any cube root function of the form, f(x) = a ∛(bx - h) + yard, just accept the same table every bit above and get new x and y-coordinates as follows according to the given part:

To become new y-coordinates., apply the outside operations of the cube root sign on the y-coordinates of the to a higher place table.
To get new x-coordinates, set bx - h equal to each of the old x-coordinates and solve for x that gives the new ten-coordinates.

Here is an example.

Instance: Graph the cube root function f(ten) = 2 ∛(x - one) + 3.

Solution:

Let us consider the old table (of parent cube root office that is mentioned above).

x	y
-8	-two
-1	-1
0	0
1	i
8	two

Let us get the new table that volition correspond to the given part in the following manner.

The new 10-coordinates can exist obtained by setting ten - 1 = erstwhile coordinate and solving for x.
The new y-coordinates can be obtained by simplifying 2(old y-coordinate) + 3.

x	y
x - ane = -8 ⇒ x = -seven	2(-2) + 3 = -1
x - 1 = -i ⇒ x = 0	ii(-i) + three = 1
10 - 1 = 0 ⇒ x = 1	ii(0) + iii = 3
x - one = 1 ⇒ x = 2	2(i) + three = 5
ten - 1 = eight ⇒ x = nine	two(2) + 3 = seven

The points from the table are (-vii, -1), (0, 1), (one, 3), (ii, 5), and (9, 7). Let us plot them, join them by a bend, and extend the curve.

graphing cube root function

Backdrop of Cube Root Office

Here are the characteristics of a cube root role f(ten) = ∛x. Nosotros can await at the graph of the parent cube root function to justify each of the following properties.

It is increasing on (-∞, ∞).
Information technology is positive on (0, ∞) and negative on (-∞, 0). f(ten) = 0 when 10 = 0.
Thus, the end behavior of the cube root office is:
f(x) → ∞ equally x → ∞
f(x) → -∞ as ten → -∞
It has no relative max/min.
It has no global max/min.
It has no asymptotes.
Its domain and range is equal to (-∞, ∞).

☛ Related Topics:

Cube Root Formula
Cube Root Calculator
Cube Root 1 to 20
Foursquare Root Formula

Cube Root Office Examples

Example 1: Which of the following are cube root functions? (a) f(x) = 3 √ten (b) f(x) 3 ∛x

Solution:

We know that a cube root function involves the cube roots in it. Thus, the respond is only (b) (as (a) represents a square root office as it involves a square root).

Answer: (b)
Example 2: Graph the function yard(10) = - ∛x + 3 using transformations.

Solution:

We take seen the graph of the parent cube root function f(ten) = ∛x on this folio. Now, our function is, g(ten) = -∛10 + 3.

Using transformations, g(x) is obtained by reflecting f(x) with respect to the ten-axis and moving it vertically up past 3 units. We can see the graphs of f(x) and g(10) in the graph beneath.

Answer: The given function is graphed using transformations.
Case 3: What is the domain and range of the function that is given in Instance ii? Does it have whatsoever asymptotes?

Solution:

We can see that the graph of g(x) = - ∛x + 3 in Instance 2 is covering the entire x and y axes. Hence, the domain and range, both are equal to the set up of all existent numbers.

Also, in the graph, we cannot see that the graph is very close to but not touching any vertical/horizontal line. Hence, information technology has no asymptotes.

Respond: Domain = Range = Gear up of all real numbers; No asymptotes.

go to slidego to slidego to slide

Take questions on basic mathematical concepts?

Go a trouble-solving gnaw using logic, non rules. Learn the why behind math with our certified experts

Book a Gratis Trial Class

Practice Questions on Cube Root Function

get to slidego to slide

FAQs on Cube Root Function

What is the Formula of Cube Root Function?

The formula for bones (parent) cube root role is f(x) = ∛ten. It can also be of the class f(x) = a ∛(bx - h) + 1000 afterwards the transformations. Here, a, b, h, and k are existent numbers and they correspond the transformations.

How to Describe Cube Root Graph?

To describe the graph of the parent cube root function f(x) = ∛x, draw a tabular array of values with ii columns x and y. Accept the numbers -8, -ane, 0, 1, and 8 in the x column (as these are perfect cubes), calculate the cube root of each of these numbers, and fill up them in the column labeled y. Then we accept 5 points (-8, -ii), (-one, -1), (0, 0), (1, 1), and (eight, two). Just plot them and join them by a curve. As this curve is not complete, just extend it on both sides throughout the graph sheet.

What is the Departure Between Foursquare Root and Cube Root Role?

Here are the differences betwixt the square root and cube root role.

Square Root Role	Cube Root Office
The basic parent square root function is of the class f(x) = √10.	The bones parent cube root function is of the class f(x) = ∛x.
It increases on the interval (-∞, ∞).	It increases on the interval [0, ∞).
It is positive on (0, ∞) and negative on (-∞, 0).	It is a not-negative function always (on [0, ∞)).
Its absolute min is 0 but no absolute max.	It has no absolute max or min.
Its domain and range is the gear up of all real numbers.	Its domain and range is [0, ∞).
Its stop behaviour is: f(x) → ∞ as 10 → ∞ f(x) → -∞ as x → -∞	Its terminate behaviour is: f(ten) → ∞ equally x → ∞ f(x) → 0 as ten → 0

How to Find the Derivative of a Cube Root Office?

The cube root role can exist written as f(ten) = ∛x = x^1/3. We can find its derivative using the ability rule of derivatives that says d/dx (x^north) = nx^{n - 1}. Using this, d/dx (x^1/3) = (ane/3) x^{(ane/3 - 1)}= (i/3) x^-ii/three = one / (3x^2/iii).

How to Integrate a Cube Root Function?

Let f(x) =∛x. It can exist written every bit ten^one/three. Its integral can be found using the formula ∫ tenⁿdx = (x^{n + 1}) / (north + 1) + C. Using this formula, ∫ x^i/3 dx = (x^{ane/3 + i}) / (ane/3 + 1) + C = (3/4) x^iv/iii + C.

What is the Domain and Range of a Cube Root Role?

The domain of a cube root office f(ten) = ∛x is the set of all real numbers (R) because information technology tin be calculated for all values of ten. Its range is also equal to the set of all real numbers because information technology volition outcome in all real numbers as y-values. In other words, the entire ten-axis and the entire y-centrality are covered by its graph and hence both domain and range are equal to R.

Does a Cube Root Function take Asymptotes?

No, a cube root function f(x) = ∛ten doesn't accept any asymptotes. Information technology doesn't have a horizontal asymptote because it is increasing on the set up of all real numbers. It doesn't have a vertical asymptote because it is divers at all existent numbers.