Graph Even Odd Or Neither
How to determine whether a part is even, odd, or neither

What are even and odd functions?
When we talk nearly "even, odd, or neither" we're talking about the symmetry of a function. It's easiest to visually run across fifty-fifty, odd, or neither when looking at a graph. Sometimes it'south hard or incommunicable to graph a function, then there is an algebraic manner to cheque besides.

Hullo! I'one thousand krista.
I create online courses to help you stone your math class. Read more.
Fifty-fifty functions
Symmetric well-nigh the ???y???-axis
When y'all plug ???-x??? into the function, it will simplify to be the same as the original function. This means that it doesn't thing whether you plug in ???x??? or ???-x???, your output will be the same. And so
???f(-x)=f(x)???
Below are graphs that are even and symmetric well-nigh the ???y???-axis.
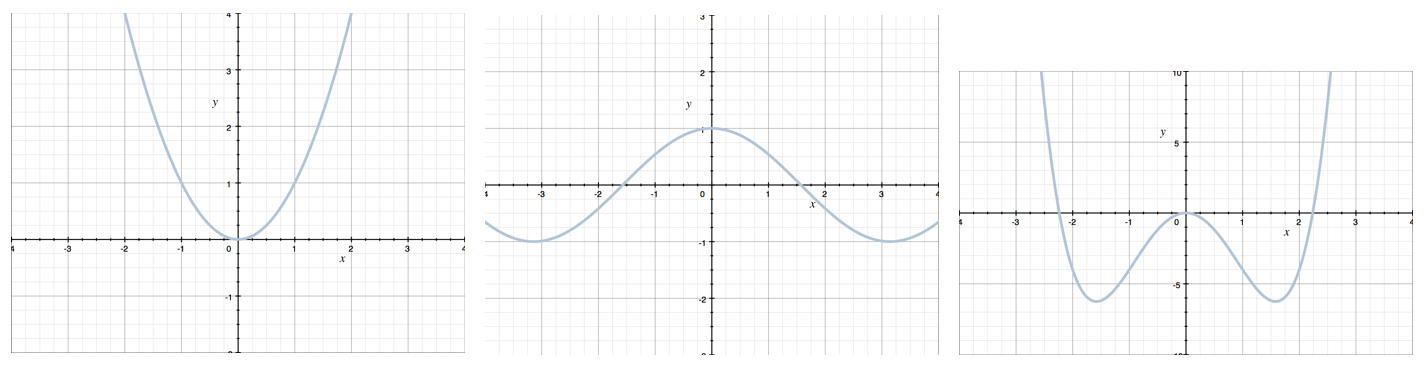
Odd functions
Symmetric about the origin
When you plug ???-x??? into the function, it volition simplify to be negative of the original part, or the original function multiplied past ???-ane???. This means that when you lot plug ???-ten??? into the function, y'all'll get the same output as you lot practise when yous plug in ???10???, except it will be negative (or take the opposite sign every bit the original output). So
???f(-x)=-f(x)???
Beneath are graphs that are odd and symmetric well-nigh the origin. Be sure to visually compare quadrants that are diagonal from each other (quadrants 1 and 3, and quadrants 2 and 4).
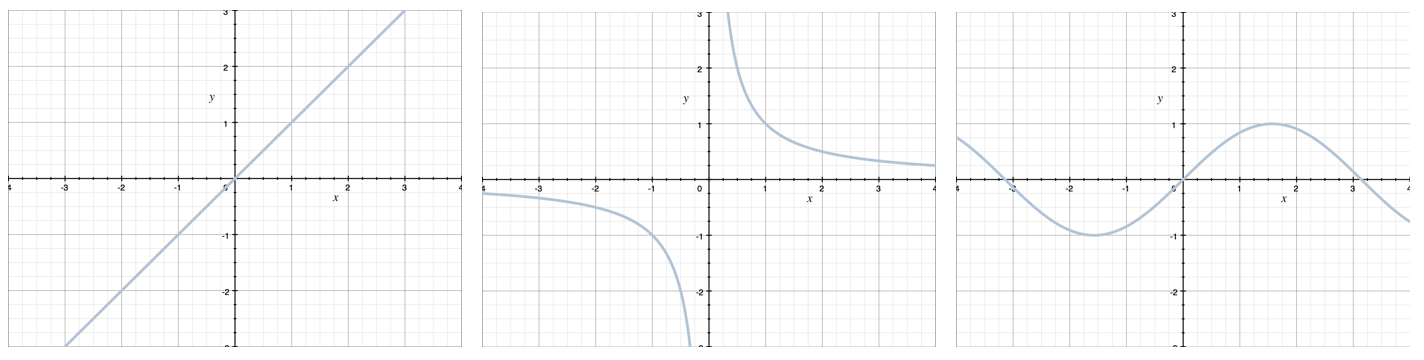
Neither fifty-fifty nor odd
Non symmetric about the ???y???-axis, and not symmetric nearly the origin
The part has no symmetry. Information technology'southward possible that a graph could be symmetric to the ???10???-axis, but then it wouldn't pass the Vertical Line Test and therefore wouldn't exist a function.
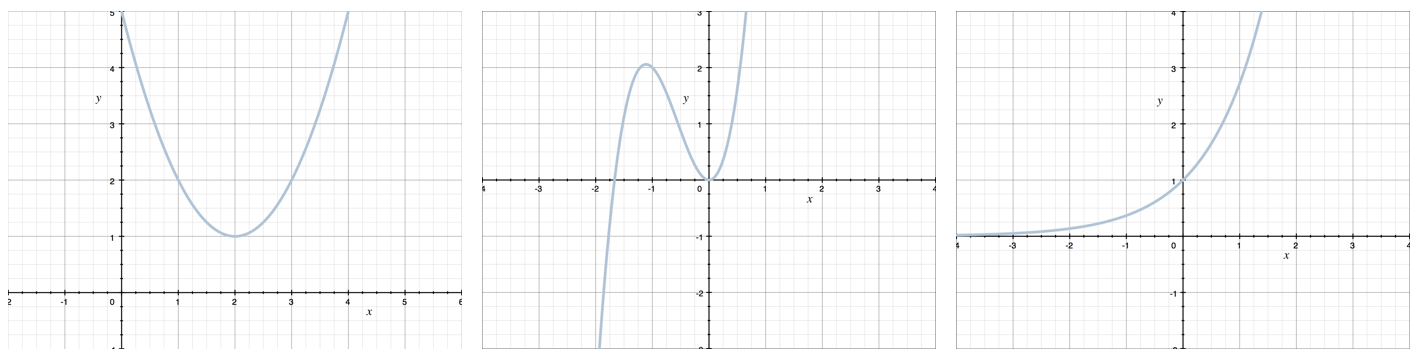
How to determine whether a function is even, odd, or neither


Have the course
Desire to learn more virtually Algebra ane? I accept a step-by-stride course for that. :)
Is the polynomial function even, odd, or neither?
Example
Is the function even, odd, or neither?
???f(x)=x^5-3x^3???
To solve algebraically we need to find ???f(-x)???, so we'll supersede all ???10???'southward with ???-x???.
???f(-x)=(-x)^five-3(-x)^three???
Raising a negative value to an odd exponent keeps the sign the same.
???f(-x)=-10^5+3x^3???
Factor out a negative.
???f(-x)=-\left(ten^v-3x^iii\right)???
Since ???f(-x)=-f(10)???, the function is odd. We tin see that the graph is symmetric to the origin.
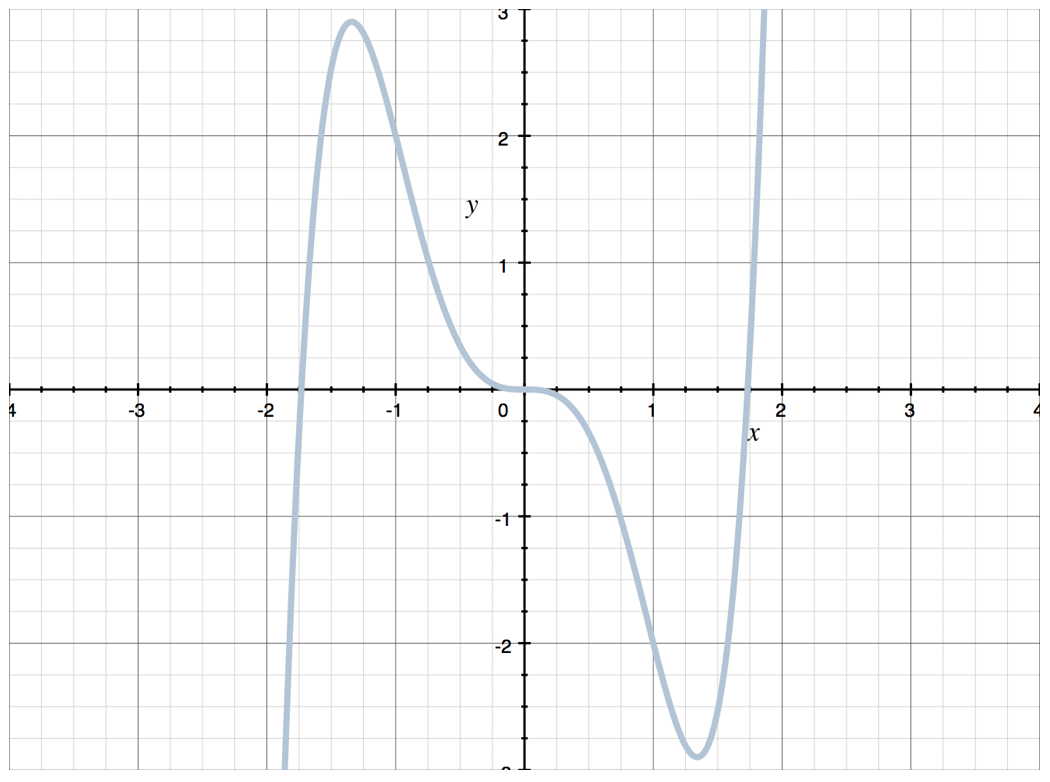
Permit's try another example of even, odd, neither.

It's easiest to visually see fifty-fifty, odd, or neither when looking at a graph.
Example
Is the part even, odd, or neither?
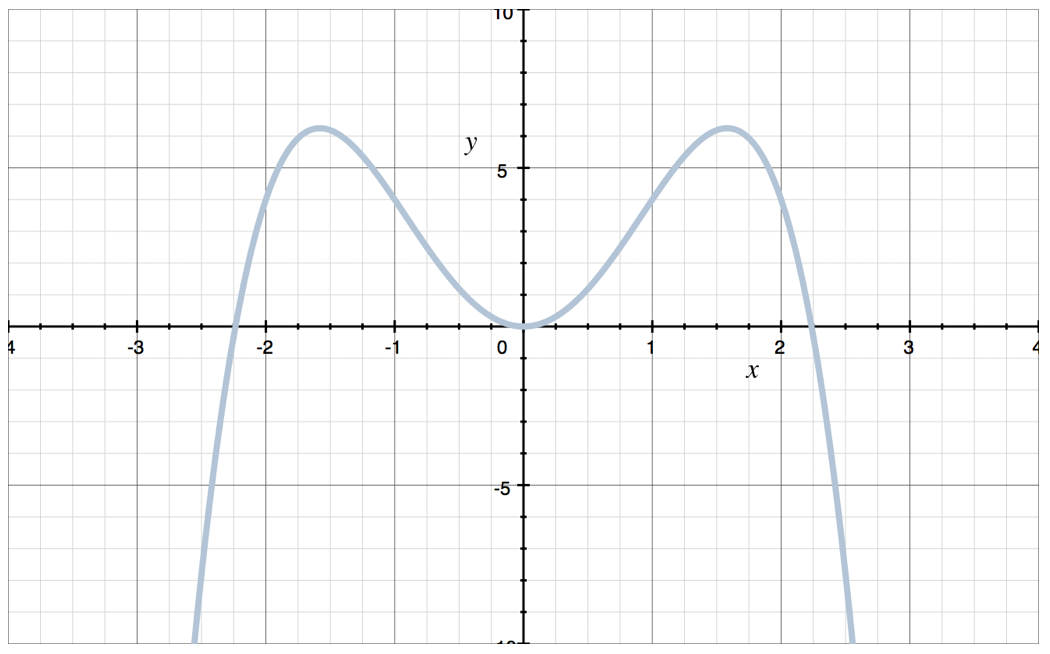
???f(x)=5x^two-10^iv???
To solve algebraically, we need to find ???f(-x)???, so we'll supersede all ???x???'s with ???-x???.
???f(-10)=five(-x)^2-(-x)^four???
Raising a negative value to an fifty-fifty exponent changes the sign.
???f(-x)=5x^2-x^iv???
Since ???f(-x)=f(10)???, the function is even. We can run across that the graph is symmetric to the ???y???-axis.

Get admission to the complete Algebra 1 class
Graph Even Odd Or Neither,
Source: https://www.kristakingmath.com/blog/even-odd-or-neither
Posted by: dentoncorties.blogspot.com


0 Response to "Graph Even Odd Or Neither"
Post a Comment